The detailed understanding of particle motion in the outer region (halo) of a bunched beam is of utmost importance for all existing and future high intensity hadron accelerators in view of minimizing particle losses and machine activation. Particle-core models separate the motion of halo particles from the core and treat them as test-particles. Therefore these reduced-order models are computationally inexpensive compared to full particle-in-cell simulations and can, to some extent, be derived analytically, thus giving insights into the non-linear mechanism of halo formation. These models have been successfully applied to linear accelerators, first by Gluckstern in 1994, for coasting round beams. The key point of Pirmin Berger's thesis is the extension of the model to ellipsoidal 3D bunched beams including dispersion, acceleration, and a self-consistent prediction of the core motion. A fully analytic model and a numerical model, the so-called extended particle-core model, have been derived. The new models were then applied to a simplified cyclotron but with parameters (e.g. tunes, energies) similar to the PSI Injector 2.
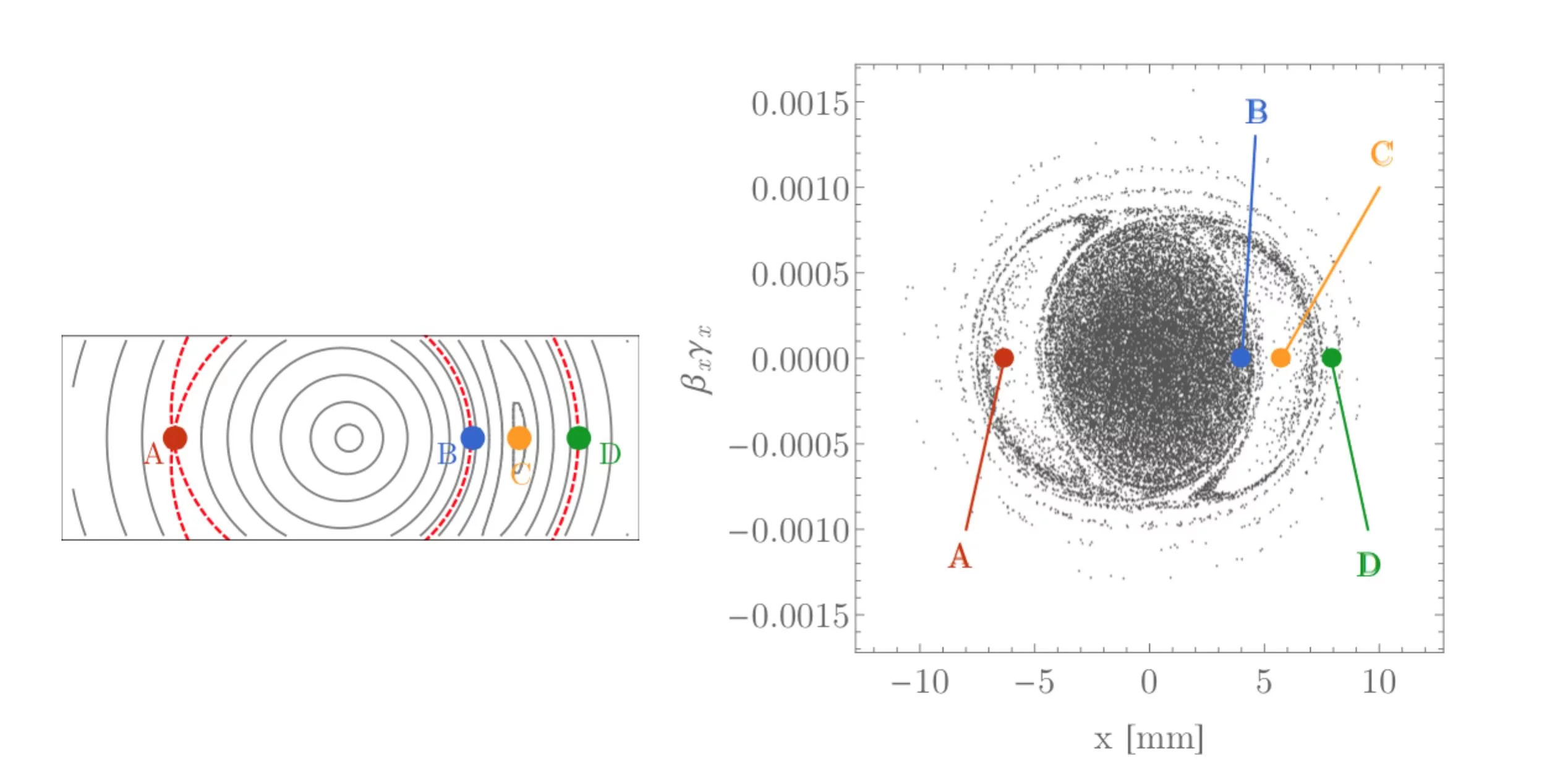
A division of the phase space into four characteristic regions (A: unstable fix-point, B: inner separatrix, C: stable fix-point and D: outer separatrix) has been observed, as depicted in Figure 1. The purely analytic model (left of Fig. 1) compares, within limits, very well to the numerical model. We speculate that such reduced-order models may be the nucleus of future on-line models, accurately describing halo phenomena in high intensity hadron accelerators such as the Injector 2 and the PSI Ring cyclotron.
A division of the phase space into four characteristic regions (A: unstable fix-point, B: inner separatrix, C: stable fix-point and D: outer separatrix) has been observed, as depicted in Figure 1. The purely analytic model (left of Fig. 1) compares, within limits, very well to the numerical model. We speculate that such reduced-order models may be the nucleus of future on-line models, accurately describing halo phenomena in high intensity hadron accelerators such as the Injector 2 and the PSI Ring cyclotron.